Education
- How Information Can Escape From A Black Hole?
Every black hole conceals a secret — the quantum remains of the star from which it formed, say a group of scientists, who also predict that these stars can later emerge once the black hole evaporates. The researchers call these objects "Planck...
- Theory Of Special Relativity
Einstein's Theory Of Special Relativity :- Special relativity is a theory of the structure of space time. It was introduced in Einstein's 1905 paper "On the Electrodynamics of Moving Bodies" . Special relativity is based on two postulates...
- What Is General Relativity?
Theory Of General Relativity:- General relativity is a theory of gravitation developed by Einstein in the years 1907–1915. The development of general relativity began with the equivalence principle, under which the states...
- Quantum Mechanics In History
Quantum Mechanics is a fundamental branch of physics with wide applications. The foundations of quantum mechanics were established during the first half of the twentieth century by Werner Heisenberg, Max Planck, Louis de Broglie, Albert Einstein, Niels...
- Brief Biography Of Albert Einstein (1879-1955)
Albert Einstein is one of the greatest scientists of all times. He questioned widely accepted scientific truths and fine-tuned the scientific theories of Sir Isaac Newton. Albert Einstein was born on March 14, 1879 in Ulm, Wurttemberg, Germany. He studied...
Education
How Quantum Randomness Saves Relativity
In physics, Albert Einstein is famous for two things: developing the theory of relativity, and hating quantum mechanics. Relativity is a colossal achievement, with the special theory of 1905 putting classical physics on a firmer philosophical footing, and the general theory of 1915 extending that idea into the best theory we have for understanding gravity. While Einstein’s “heuristic” model of the photoelectric effect, also from 1905, played a critical role in launching quantum physics, he ended up finding it distasteful on the same sort of philosophical grounds that made relativity so successful. In particular, he hated the indeterminacy of the full quantum theory as it developed in the 1920′s. The notion that quantum particles do not have well-defined states prior to measurement was just too radical a departure from classical physics for Einstein to stomach, and he spent the last decades of his life searching for a replacement theory that would be more philosophically congenial.
 There’s no small irony, then, in the fact that the very randomness he disparaged with his famous line about refusing to believe that God plays dice with the universe (paraphrased lots of times, but originally from a letter to Max Born) is essential for preserving one of the key results of his theory of relativity. Without that indeterminacy, quantum physics would allow the sending of messages faster than the speed of light, with disastrous consequences for the whole idea of causality, which is central to the operation of physics.
There’s no small irony, then, in the fact that the very randomness he disparaged with his famous line about refusing to believe that God plays dice with the universe (paraphrased lots of times, but originally from a letter to Max Born) is essential for preserving one of the key results of his theory of relativity. Without that indeterminacy, quantum physics would allow the sending of messages faster than the speed of light, with disastrous consequences for the whole idea of causality, which is central to the operation of physics.
How does this work out? Well, the key phenomenon is the idea we now know as “entanglement,” a phenomenon that Einstein was concerned with back in the 1920′s when he was famously arguing with Niels Bohr (I re-enacted this with puppets some years back). Its clearest expression came in a 1935 paper by Einstein with his young colleagues Boris Podolsky and Nathan Rosen (colloquially known as the “EPR paper” after the initials of the authors), in which they used entanglement to argue that quantum physics as then understood must be an incomplete version of a deeper, more deterministic theory.
While the EPR paper talks in terms of position and momentum, it’s easiest to understand the physics of entanglement in terms of two-state variables like polarization of photons. (This version gets its own collection of initials, “CHSH” for John Clauser, Mike Horne, Abner Shimony, and Richard Holt who worked it our in the late 1960′s.) If you have the right sort of light source, either a single highly excited atom or a crystal of the right material, you can produce pairs of photons that do not have a definite polarization until they are measured, but that will always have correlated polarizations.
If you take two such photons and send them to two different scientists to measure– traditionally, they’re named Alice and Bob– you can demonstrate that these correlations do not seem to respect Einstein’s relativity. That is, if Alice measures her photon in New York at precisely noon, and finds that it has vertical polarization, then Bob measures his a nanosecond later in Paris, he will also find that it has vertical polarization. In order for Bob’s measurement to perfectly match Alice’s, either the outcome of both measurements must be determined in advance (the preferred model of the original EPR authors), or some sort of signal must be passing from Alice to Bob at speeds vastly in excess of the speed of light. This latter possibility is the phenomenon that Einstein dismissed as “spukhafte fernwirkung,” “spooky action at a distance.” It’s a bit of supernatural activity that he felt could not have any place in a sensible theory of physics.
Of course, in 1965 John Bell showed that the determined-in-advance sort of models Einstein would’ve preferred would be bound by limits that quantum physics could exceed. Experimental tests in the 1970′s and early 1980′s showed that quantum reality does, in fact, exceed those limits. The state of Bob’s photon really is correlated with Alice’s measurement outcome in a way that can not possible be explained by a model where both photons have predetermined states. This has been confirmed in countless experiments since, and these days the technology required is well within the reach of an undergraduate laboratory.
 This might seem like it opens the possibility of faster-than light communication between Alice and Bob. They simply share entangled photons with each other, and then measure their polarizations, calling one outcome “0″ and the other “1.” This lets them transmit messages in binary code, and violate the restriction from relativity that nothing can exceed the speed of light.
This might seem like it opens the possibility of faster-than light communication between Alice and Bob. They simply share entangled photons with each other, and then measure their polarizations, calling one outcome “0″ and the other “1.” This lets them transmit messages in binary code, and violate the restriction from relativity that nothing can exceed the speed of light.
But this is where quantum randomness, the divine dice-throwing that Einstein derided, steps in to save the day. The simplest possible scheme you could imagine– Alice assigns a “1″ to vertical polarization and a “0″ to horizontal polarization– doesn’t work at all, because there’s no way to force a particular outcome. When Alice sets her polarization analyzer to vertical, there’s a 50% chance that her photon will turn out vertical, and a 50% probability that it turns out horizontal. Bob’s photons will be perfectly correlated with Alice’s, but all that means is that they each have the same totally random string of 0′s and 1′s. No information passes from Alice to Bob.
You could imagine doing a slightly more sophisticated thing, though, and assigning “1″ to Alice making her measurement checking for either horizontal or vertical, and “0″ to making her measurement at a 45 degree angle (up-and-right versus up-and-left). The vertical and diagonal measurement bases will show the same correlation– if Alice measures vertical, Bob’s photon will also be vertical, and if Alice measures up-and-right, Bob’s photon will also be up-and-right– but they’re potentially distinguishable from each other.
The question, though, is how do you distinguish between these? For that, we have to think about what happens when you send diagonally polarized light at a polarizing filter. It turns out that a diagonally polarized photon has a 50% chance of passing a vertical filter and vice-versa. So, if Alice’s polarizer is set to up-and-right, Bob has a 50% chance of detecting it as vertical, and a 50% chance of detecting it as horizontal.
(You can easily demonstrate this for yourself if you have an LCD monitor (which uses the polarization of light to switch pixels on and off) and a pair of polarizing sunglasses. If you hold the glasses up in front of the monitor and turn them around, you’ll find some angle where the glasses look completely black, and no light makes it through. 90 degrees away from that, there’s an angle where the image through the glasses is as bright as it gets (the axis of the glasses is aligned perfectly with the axis of the output polarizer for the monitor). Halfway between, the image is, well, halfway bright– 50% of the light gets through the glasses, and 50% gets blocked.)
So, if Alice and Bob want to send messages faster than light, Alice just varies the setting of her polarizer, while Bob keeps his set to look for vertical and horizontal. When Alice’s photon comes in with a 50/50 chance of passing the polarizer, Bob knows to call that a “0,” otherwise he marks it down as a “1.” And then they’re passing real information back and forth.
Except, that doesn’t work either, thanks to quantum randomness. There’s no way to get a probability out of a single measurement, after all– you just get a single click. If you want a probability distribution, you need to do a lot of measurements and use them to infer the probability (by either frequentist or Bayesian means, as you like). But Alice can’t just repeat the same setting 100 times for each bit she wants to send, because even if she’s sending a “1″ by putting her polarization analyzer vertical, Bob will detect roughly 50 of those as vertical and 50 of those as horizontal, exactly as would be the case for a diagonal polarization. Bob ends up with no actual information from Alice.

Portrait of Einstein by Alice Reischer, based on a sketch made during a 1939 lecture. Hangs in the Department of Physics and Astronomy at Union College, a gift of Carl George. (Photo by Chad Orzel)
How does this work out? Well, the key phenomenon is the idea we now know as “entanglement,” a phenomenon that Einstein was concerned with back in the 1920′s when he was famously arguing with Niels Bohr (I re-enacted this with puppets some years back). Its clearest expression came in a 1935 paper by Einstein with his young colleagues Boris Podolsky and Nathan Rosen (colloquially known as the “EPR paper” after the initials of the authors), in which they used entanglement to argue that quantum physics as then understood must be an incomplete version of a deeper, more deterministic theory.
While the EPR paper talks in terms of position and momentum, it’s easiest to understand the physics of entanglement in terms of two-state variables like polarization of photons. (This version gets its own collection of initials, “CHSH” for John Clauser, Mike Horne, Abner Shimony, and Richard Holt who worked it our in the late 1960′s.) If you have the right sort of light source, either a single highly excited atom or a crystal of the right material, you can produce pairs of photons that do not have a definite polarization until they are measured, but that will always have correlated polarizations.
If you take two such photons and send them to two different scientists to measure– traditionally, they’re named Alice and Bob– you can demonstrate that these correlations do not seem to respect Einstein’s relativity. That is, if Alice measures her photon in New York at precisely noon, and finds that it has vertical polarization, then Bob measures his a nanosecond later in Paris, he will also find that it has vertical polarization. In order for Bob’s measurement to perfectly match Alice’s, either the outcome of both measurements must be determined in advance (the preferred model of the original EPR authors), or some sort of signal must be passing from Alice to Bob at speeds vastly in excess of the speed of light. This latter possibility is the phenomenon that Einstein dismissed as “spukhafte fernwirkung,” “spooky action at a distance.” It’s a bit of supernatural activity that he felt could not have any place in a sensible theory of physics.
Of course, in 1965 John Bell showed that the determined-in-advance sort of models Einstein would’ve preferred would be bound by limits that quantum physics could exceed. Experimental tests in the 1970′s and early 1980′s showed that quantum reality does, in fact, exceed those limits. The state of Bob’s photon really is correlated with Alice’s measurement outcome in a way that can not possible be explained by a model where both photons have predetermined states. This has been confirmed in countless experiments since, and these days the technology required is well within the reach of an undergraduate laboratory.

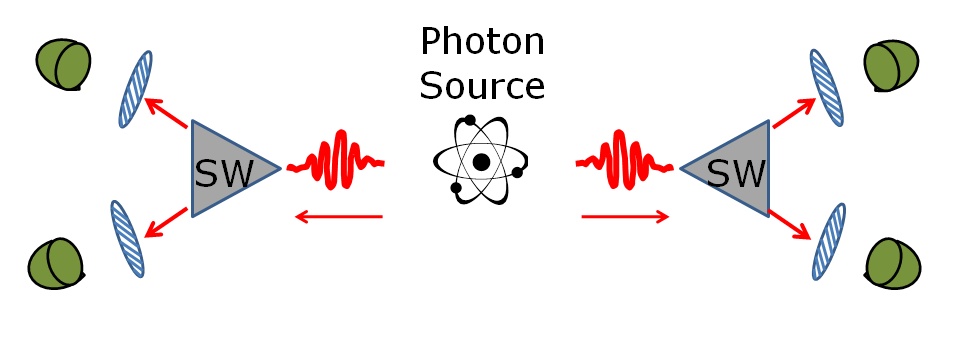
Schematic of the third Aspect experiment testing quantum non-locality. Entangled photons from the source are sent to two fast switches, that direct them to polarizing detectors. The switches change settings very rapidly, effectively changing the detector settings for the experiment while the photons are in flight. (Figure by Chad Orzel)
But this is where quantum randomness, the divine dice-throwing that Einstein derided, steps in to save the day. The simplest possible scheme you could imagine– Alice assigns a “1″ to vertical polarization and a “0″ to horizontal polarization– doesn’t work at all, because there’s no way to force a particular outcome. When Alice sets her polarization analyzer to vertical, there’s a 50% chance that her photon will turn out vertical, and a 50% probability that it turns out horizontal. Bob’s photons will be perfectly correlated with Alice’s, but all that means is that they each have the same totally random string of 0′s and 1′s. No information passes from Alice to Bob.
You could imagine doing a slightly more sophisticated thing, though, and assigning “1″ to Alice making her measurement checking for either horizontal or vertical, and “0″ to making her measurement at a 45 degree angle (up-and-right versus up-and-left). The vertical and diagonal measurement bases will show the same correlation– if Alice measures vertical, Bob’s photon will also be vertical, and if Alice measures up-and-right, Bob’s photon will also be up-and-right– but they’re potentially distinguishable from each other.
The question, though, is how do you distinguish between these? For that, we have to think about what happens when you send diagonally polarized light at a polarizing filter. It turns out that a diagonally polarized photon has a 50% chance of passing a vertical filter and vice-versa. So, if Alice’s polarizer is set to up-and-right, Bob has a 50% chance of detecting it as vertical, and a 50% chance of detecting it as horizontal.
(You can easily demonstrate this for yourself if you have an LCD monitor (which uses the polarization of light to switch pixels on and off) and a pair of polarizing sunglasses. If you hold the glasses up in front of the monitor and turn them around, you’ll find some angle where the glasses look completely black, and no light makes it through. 90 degrees away from that, there’s an angle where the image through the glasses is as bright as it gets (the axis of the glasses is aligned perfectly with the axis of the output polarizer for the monitor). Halfway between, the image is, well, halfway bright– 50% of the light gets through the glasses, and 50% gets blocked.)
So, if Alice and Bob want to send messages faster than light, Alice just varies the setting of her polarizer, while Bob keeps his set to look for vertical and horizontal. When Alice’s photon comes in with a 50/50 chance of passing the polarizer, Bob knows to call that a “0,” otherwise he marks it down as a “1.” And then they’re passing real information back and forth.
Except, that doesn’t work either, thanks to quantum randomness. There’s no way to get a probability out of a single measurement, after all– you just get a single click. If you want a probability distribution, you need to do a lot of measurements and use them to infer the probability (by either frequentist or Bayesian means, as you like). But Alice can’t just repeat the same setting 100 times for each bit she wants to send, because even if she’s sending a “1″ by putting her polarization analyzer vertical, Bob will detect roughly 50 of those as vertical and 50 of those as horizontal, exactly as would be the case for a diagonal polarization. Bob ends up with no actual information from Alice.
If you want to use Alice’s polarization measurement to send a message to Bob, what you need is to clone Bob’s photon– make a hundred prefect copies of that one photon, measure them all, and get the probability that way. That would let Bob distinguish diagonal from vertical polarization, and extract Alice’s message. But relativity is again saved by the mathematics of quantum physics– in 1982, Bill Wootters (one of my undergrad professors) and Wojciech Zurek proved a “no-cloning theorem”, which forbids the exact operation you would need to send information faster than light using entangled photons. The proof is surprisingly simple and powerful– just a few lines of algebra, see this quantum wiki or a write-up by Wootters and Zurek (pdf)– and applies to any arbitrary quantum system. If you know in advance what the state of a system is, you can make many copies (this is essentially how a laser works, using stimulated emission to make many identical photons), but an indeterminate state cannot be faithfully duplicated. Any attempt to copy the state of Bob’s photon will necessarily introduce random noise that destroys the attempt to determine the polarization.
So, for all Einstein’s complaints about the probabilistic nature of quantum physics, it’s that very randomness that serves to protect relativity. Which is very good for those of us who like causes to precede effects, and also an enjoyable bit of historical irony.
- How Information Can Escape From A Black Hole?
Every black hole conceals a secret — the quantum remains of the star from which it formed, say a group of scientists, who also predict that these stars can later emerge once the black hole evaporates. The researchers call these objects "Planck...
- Theory Of Special Relativity
Einstein's Theory Of Special Relativity :- Special relativity is a theory of the structure of space time. It was introduced in Einstein's 1905 paper "On the Electrodynamics of Moving Bodies" . Special relativity is based on two postulates...
- What Is General Relativity?
Theory Of General Relativity:- General relativity is a theory of gravitation developed by Einstein in the years 1907–1915. The development of general relativity began with the equivalence principle, under which the states...
- Quantum Mechanics In History
Quantum Mechanics is a fundamental branch of physics with wide applications. The foundations of quantum mechanics were established during the first half of the twentieth century by Werner Heisenberg, Max Planck, Louis de Broglie, Albert Einstein, Niels...
- Brief Biography Of Albert Einstein (1879-1955)
Albert Einstein is one of the greatest scientists of all times. He questioned widely accepted scientific truths and fine-tuned the scientific theories of Sir Isaac Newton. Albert Einstein was born on March 14, 1879 in Ulm, Wurttemberg, Germany. He studied...
